Optimization
Intelligent optimization is an area that creates much value for the customers. The most common question that is answered is probably “How should the product be design to minimize the material cost with kept performance?”, but there are a number of other questions that also can be answered. In the example below an optimization of a manufacturing tool has been performed in order to reduce problems related to the manufacturing process.
Optimization example
Below is a presentation of a simple optimization of a creasing tool used in the paper packaging industry. When paperboard is folded into packages the board first needs to be creased so that the subsequent folding will give a well defined edge, this is done with the creasing tool.
Underlining problem: There is a risk that the paperboard cracks when the board is creased and folded.
Objectives: Minimize the risk of board fracture during creasing and minimize the risk of board fracture during folding.
Parameters: In the optimization five parameters are altered, as example the creasing depth.
Results: Since there are two objectives in the optimization there is not a single best design of the manufacturing tool, instead there is a front, called the Pareto frontier, in two dimensions which are the best designs. In the figure below the results of the optimization is shown. Each dot represents a simulation, and each axis represents an objective. The optimization algorithm strives to give the tool a shape that lower the risk of fracture and drive the simulations towards the lower left side of the diagram.
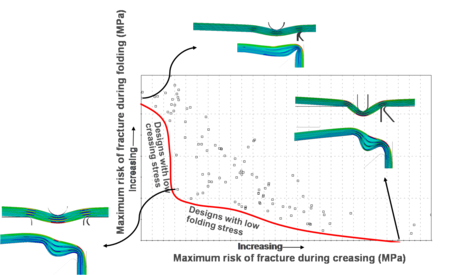
Representation of simulations visualized towards the objectives of the optimization. The aim is the lower left corner of the graph. This gives the best designs (the Pareto frontier) following the red line. Pictures from the simulations are taken at the end of the creasing operation and the end of the folding operation.